Solution: 2015 Fall Midterm - 16

Author: Michiel Smid

Question

You roll a fair 6-sided die twice. Define the events

and

Which of the following is true?

(a)

None of the above.

(b)

$\Pr(A) = \Pr(B)$

(c)

$\Pr(A) > \Pr(B)$

(d)

$\Pr(A) < \Pr(B)$

For this question, we can draw a table to see the possible outcomes.

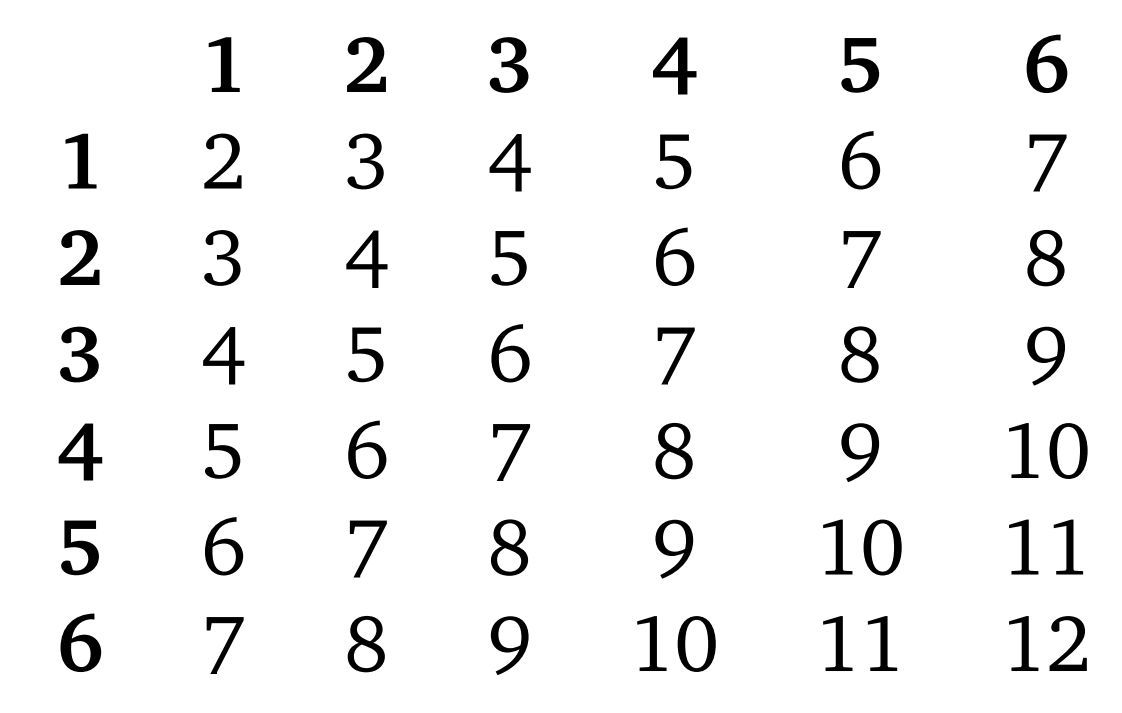
A total of 7 can be obtained by 6 combinations: (1,6), (2,5), (3,4), (4,3), (5,2), (6,1)

$|A|=6$

$ Pr(A) = \frac{6}{36} = \frac{1}{6}$

Out of 36 possibilities, a 3 on the first roll can be obtained 6 times: (3,1), (3,2), (3,3), (3,4), (3,5), (3,6)

$|B|=6$

$ Pr(B) = \frac{6}{36} = \frac{1}{6}$

$ Pr(A)=Pr(B)$